Plane geo
-
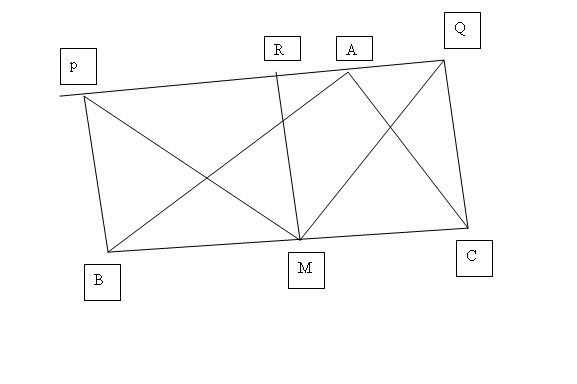
A line PQ is drawn through the verex A of triangle ABC such that BP and CQ are perpendicular to PQ. If M is the midpoint of BC and MR is perpendicular to PQ, prove that MP = MQ.
My concept is that since the angles BPR, MRP, MRQ, CQR are all perpendicular, this renders BC to be equal to PQ as angles BMR, MCQ are also 90 degrees(parallel lines, corres. angles). Since BC = PQ, and BM = MC, PQ = RQ and this trangle PRM is isoceles. so MP = MQ.
Feels like there's something missing in my proving. Can someone verify? thanks
-
Originally posted by bonkysleuth:
A line PQ is drawn through the verex A of triangle ABC such that BP and CQ are perpendicular to PQ. If M is the midpoint of BC and MR is perpendicular to PQ, prove that MP = MQ.
My concept is that since the angles BPR, MRP, MRQ, CQR are all perpendicular, this renders BC to be equal to PQ as angles BMR, MCQ are also 90 degrees(parallel lines, corres. angles). Since BC = PQ, and BM = MC, PQ = RQ and this trangle PRM is isoceles. so MP = MQ.
Feels like there's something missing in my proving. Can someone verify? thanks

Spotted a mistake, PQ=RQ. It should be PR = RQ.
If you ask me, RM, PB and QC are perpendicular to PQ, M is the center of BC, hence R is the midpoint of PQ.
= > PRMB = RQCM
= > MP = MQ
Hope this helps.
-
ok i would prove it this way
as per the question, BP and CQ are both perpendiular to PQ. Thus they are parallel. Thus BCQP is a trapezium
M is the midpoint of BC and MR is perpendicular to PQ. Then PR = RQ. Thus MR^2+PR^2 = MR^2+RQ^2 and hence MP = MQ
-
I think I saw this question in an assessment book before
Answer is because BM = MC, hence PR = RQ
And since MR is perpendicular to PQ, then triangle MPQ is an isosceles triangle, hence MP = MQ
or as jay put it
Then PR = RQ. Thus MR^2+PR^2 = MR^2+RQ^2 and hence MP = MQ
-
I just read through the following sentences which are untrue and wrong.
My concept is that since the angles BPR, MRP, MRQ, CQR are all perpendicular, this renders BC to be equal to PQ as angles BMR, MCQ are also 90 degrees
= > PRMB = RQCM